Next: Hydrogen Atom in a Up: Fine Structure in Hydrogen Previous: Fine Structure in Hydrogen Contents
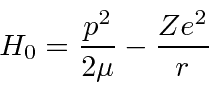
To this simple Coulomb problem, we will add several corrections:
Correction (1) comes from relativity.
The electron's velocity in hydrogen is of order
![]() .
It is not very relativistic but a small correction is in order.
By calculating
the next order relativistic correction to the kinetic energy
we find the additional term in the Hamiltonian
.
It is not very relativistic but a small correction is in order.
By calculating
the next order relativistic correction to the kinetic energy
we find the additional term in the Hamiltonian
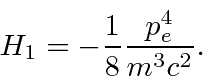
We can estimate the size of this correction compared to the Hydrogen binding energy by taking the
ratio to the Hydrogen kinetic energy.
(Remember that, in the hydrogen ground state,
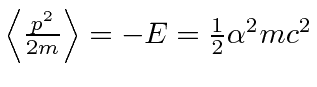 .)
.)
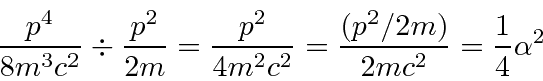
The second term, due to Spin-Orbit interactions, is harder to derive correctly. We understand the basis for this term. The magnetic moment from the electron's spin interacts with the B field produced by the current seen in the electron's rest frame from the circulating proton.
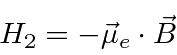
We can derive
B from a Lorentz transformation of the E field of a static proton
(We must also add in the Thomas Precession which we will not try to understand here).
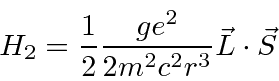
Now we
compute
the relativity correction in first order perturbation theory .
![\begin{displaymath}\bgroup\color{black} \left< \psi_{nlm}\left\vert H_1\right\ve...
...mc^2}} \left[ 3 - {4n \over{\ell + {1\over 2}}} \right] \egroup\end{displaymath}](img3075.png)
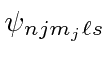 or the
or the
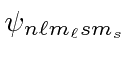 to calculate the
effect of
to calculate the
effect of
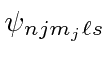 to add in the spin-orbit.
to add in the spin-orbit.
The first order perturbation energy shift from the spin orbit correction is
calculated
for the states of definite ![]() .
.
![\begin{displaymath}\bgroup\color{black}\left<\psi_{nlm}\left\vert H_2\right\vert...
...l(\ell+1)-s(s+1)\right]\left<{1\over{r^3}}\right>_{nlm} \egroup\end{displaymath}](img3078.png)
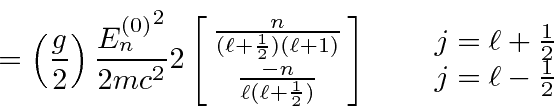
Actually, the
![]() term should give 0 for
term should give 0 for
![]() !
In the above calculation there is an
!
In the above calculation there is an
![]() factor which makes the result for
factor which makes the result for
![]() undefined.
There is an additional Dirac Equation contribution called the ``Darwin term''
which is important for
undefined.
There is an additional Dirac Equation contribution called the ``Darwin term''
which is important for
![]() and surprisingly makes the above calculation right, even for
and surprisingly makes the above calculation right, even for
![]() !
!
We will now add these three fine structure corrections together for states of definite
![]() .
We start with a formula which has slightly different forms for
.
We start with a formula which has slightly different forms for
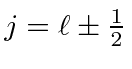 .
.
![\begin{displaymath}\bgroup\color{black}E_{njm_j\ell s}=E^{(0)}_n + {{E^{(0)}_n}^...
...{\ell(\ell+{1\over 2})}} }
\right\}^{(+)}_{(-)}\right]\egroup\end{displaymath}](img3082.png)
![\begin{displaymath}\bgroup\color{black}E_{njm_j\ell s} =E^{(0)}_n + {{E^{(0)}_n}...
...ll+1}} \cr 4+{2\over\ell}}
\right\}^{(+)}_{(-)}\right]\egroup\end{displaymath}](img3083.png)
![\begin{displaymath}\bgroup\color{black}E_{njm_j\ell s} =E^{(0)}_n + {{E^{(0)}_n}...
...{2\over{j+{1\over 2}}}\over{\ell+{1\over 2}} }
\right]\egroup\end{displaymath}](img3084.png)
We can write
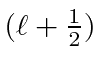 as
as
 , so that
, so that

![\bgroup\color{black}$E_{nlm} = E^{(0)}_n
+ {{E^{(0)}_n}^2 \over {2mc^2}} \left[3 - {4n\over{j+{1\over 2}}}\right]$\egroup](img3088.png) |

We have calculated the fine structure effects in Hydrogen.
There are, of course, other, smaller corrections to the energies.
A correction from field theory, the Lamb Shift, causes states of different
![]() to shift apart slightly.
Nevertheless, the states of definite total angular momentum are the energy eigenstates until we somehow break
spherical symmetry.
to shift apart slightly.
Nevertheless, the states of definite total angular momentum are the energy eigenstates until we somehow break
spherical symmetry.
Jim Branson 2013-04-22