- Calculate the lowest order energy shift for the (0th order degenerate first)
excited states of Helium
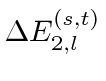 where
where  .
This problem is set up in the
discussion of the first excited states.
The following formulas will aid you in the computation.
First, we can expand the formula for the inverse distance between the two electrons as follows.
.
This problem is set up in the
discussion of the first excited states.
The following formulas will aid you in the computation.
First, we can expand the formula for the inverse distance between the two electrons as follows.
Here 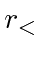 is the smaller of the two radii and
is the smaller of the two radii and 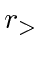 is the larger.
As in the ground state calculation, we can use the symmetry of the problem to specify which radius is the larger.
Then we can use a version of the addition theorem to write the Legendre Polynomial
is the larger.
As in the ground state calculation, we can use the symmetry of the problem to specify which radius is the larger.
Then we can use a version of the addition theorem to write the Legendre Polynomial
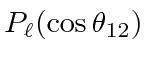 in terms of
the spherical hamonics for each electron.
in terms of
the spherical hamonics for each electron.
Using the equation
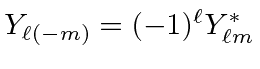 , this sets us up to do our integrals nicely.
, this sets us up to do our integrals nicely.
- Consider the lowest state of ortho-helium. What is the magnetic
moment? That is what is the interaction with an external magnetic
field?
- A proton and neutron are bound together into a deuteron, the nucleus of
an isotope of hydrogen. The binding energy is found to be -2.23 MeV for the
nuclear ground state, an
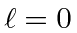 state.
Assuming a potential of the form
state.
Assuming a potential of the form
 ,
with
,
with 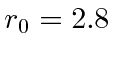 Fermis,
use the variational principle to estimate the strength of the potential.
Fermis,
use the variational principle to estimate the strength of the potential.
- Use the variational principle with a gaussian trial wave function to
prove that a one dimensional attractive potential will always have a bound
state.
- Use the variational principle to estimate the ground state energy
of the anharmonic oscillator,
 .
.
Jim Branson
2013-04-22
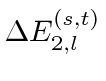 where
where  .
This problem is set up in the
discussion of the first excited states.
The following formulas will aid you in the computation.
First, we can expand the formula for the inverse distance between the two electrons as follows.
.
This problem is set up in the
discussion of the first excited states.
The following formulas will aid you in the computation.
First, we can expand the formula for the inverse distance between the two electrons as follows.
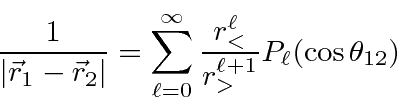
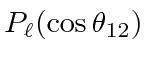 in terms of
the spherical hamonics for each electron.
in terms of
the spherical hamonics for each electron.

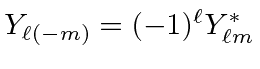 , this sets us up to do our integrals nicely.
, this sets us up to do our integrals nicely.
 ,
with
,
with 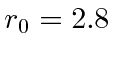 Fermis,
use the variational principle to estimate the strength of the potential.
Fermis,
use the variational principle to estimate the strength of the potential.
 .
.