- We wish to get a good upper limit on the Helium ground state energy.
Use as a trial wave function the 1s hydrogen state with the parameter
a screened nuclear charge
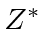 to get this limit.
Determine the value of
to get this limit.
Determine the value of 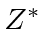 which gives the best limit.
The integral
which gives the best limit.
The integral
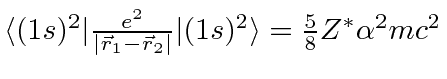 for a nucleus of charge
for a nucleus of charge  .
.
- A Helium atom has two electrons bound to a
 nucleus.
We have to add the coulomb repulsion term (between the two electrons) to the zeroth order
Hamiltonian.
nucleus.
We have to add the coulomb repulsion term (between the two electrons) to the zeroth order
Hamiltonian.
The first excited state of Helium has one electron in the 1S state and the other in the 2S state.
Calculate the energy of this state to zeroth order in the perturbation V.
Give the answer in eV.
The spins of the two electrons can be added to give states of total spin  .
The possible total spin states are
.
The possible total spin states are  and
and 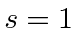 .
Write out the full first excited Helium state which has
.
Write out the full first excited Helium state which has 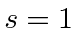 and
and 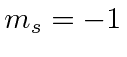 .
Include the spatial wave function and don't forget the Pauli principle.
Use bra-ket notation to calculate the energy shift to this state in first order perturbation theory.
Don't do any integrals.
.
Include the spatial wave function and don't forget the Pauli principle.
Use bra-ket notation to calculate the energy shift to this state in first order perturbation theory.
Don't do any integrals.
Jim Branson
2013-04-22
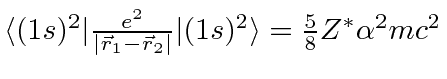 for a nucleus of charge
for a nucleus of charge 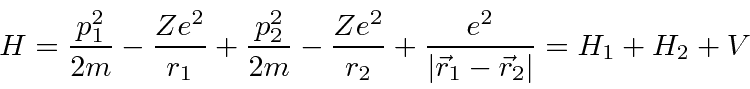
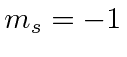 .
Include the spatial wave function and don't forget the Pauli principle.
Use bra-ket notation to calculate the energy shift to this state in first order perturbation theory.
Don't do any integrals.
.
Include the spatial wave function and don't forget the Pauli principle.
Use bra-ket notation to calculate the energy shift to this state in first order perturbation theory.
Don't do any integrals.