Next: The Variational Principle (Rayleigh-Ritz Up: The Helium Atom Previous: The Helium Ground State Contents

It's easy to show that
 .
Therefore, the spin triplet energy is lower.
We can write the energy in terms of the Pauli matrices:
.
Therefore, the spin triplet energy is lower.
We can write the energy in terms of the Pauli matrices:
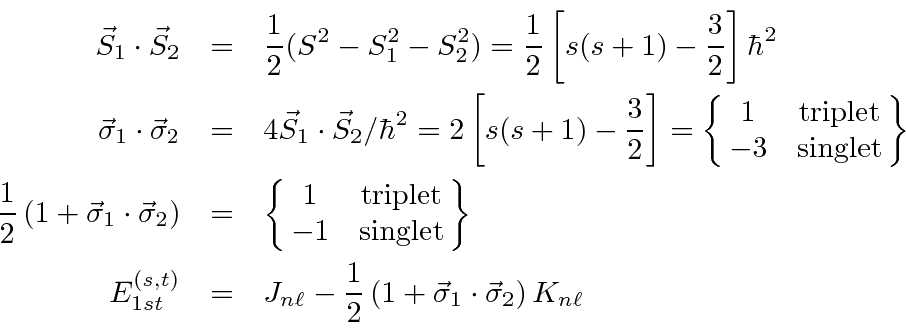
Thus we have a large effective spin-spin interaction entirely due to electron repulsion. There is a large difference in energy between the singlet and triplet states. This is due to the exchange antisymmetry and the effect of the spin state on the spatial state (as in ferromagnetism).
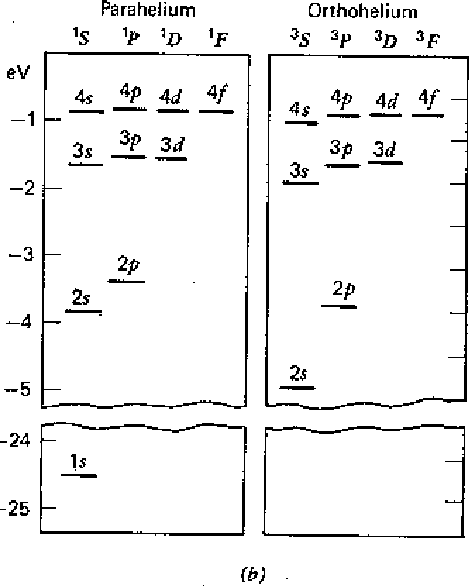
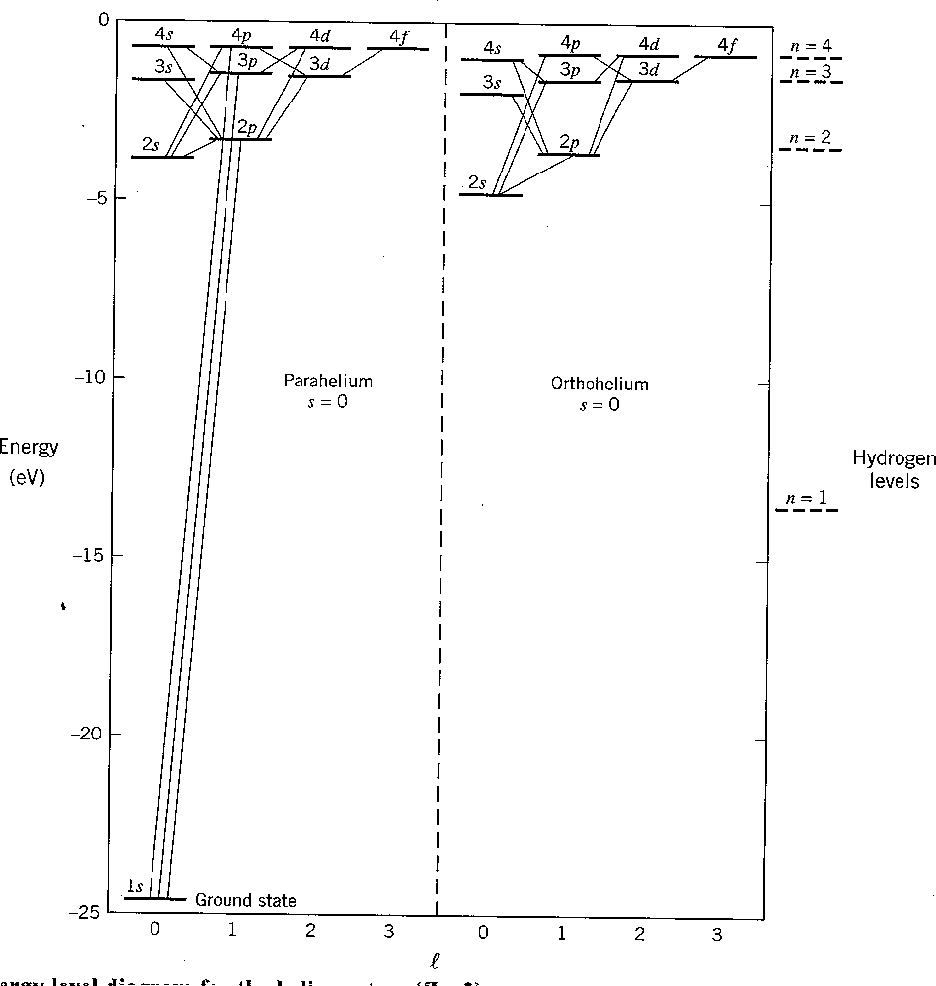
The first diagram below shows the result of our calculation. All states increase in energy due to the Coulomb repulsion of the electrons. Before the perturbation, the first excited state is degenerate. After the perturbation, the singlet and triplet spin states split significantly due to the symmetry of the spatial part of the wavefunction. We designate the states with the usual spectroscopic notation.
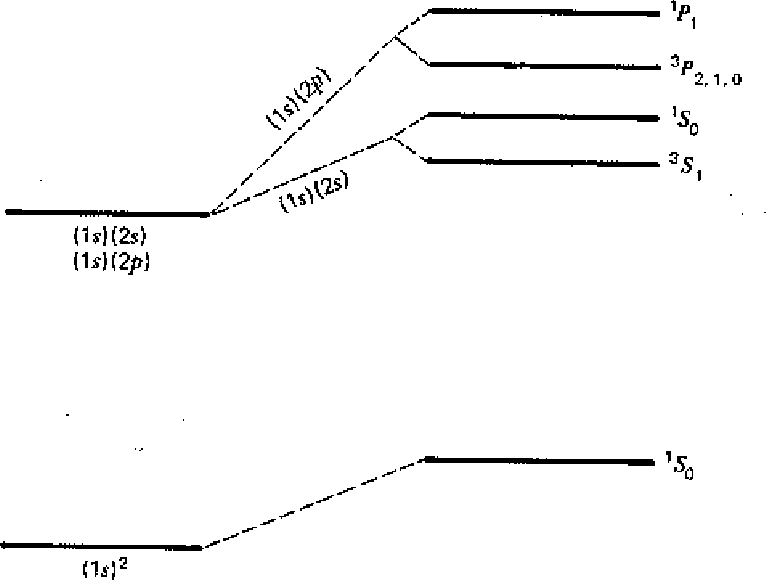
In addition to the large energy shift between the singlet and triplet states, Electric Dipole decay selection rules
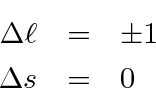
Jim Branson 2013-04-22