The Expected Velocity and Zitterbewegung

The expected value of the velocity in a plane wave state can be simply calculated.

$$\begin{eqnarray*} \langle v_k\rangle=\int\psi^\dagger (ic\gamma_4\gamma_k)\psi ... ...mc^2}={p_xc\over EV}c \\ \langle v_k\rangle={p_kc^2\over E} \\ \end{eqnarray*}$$

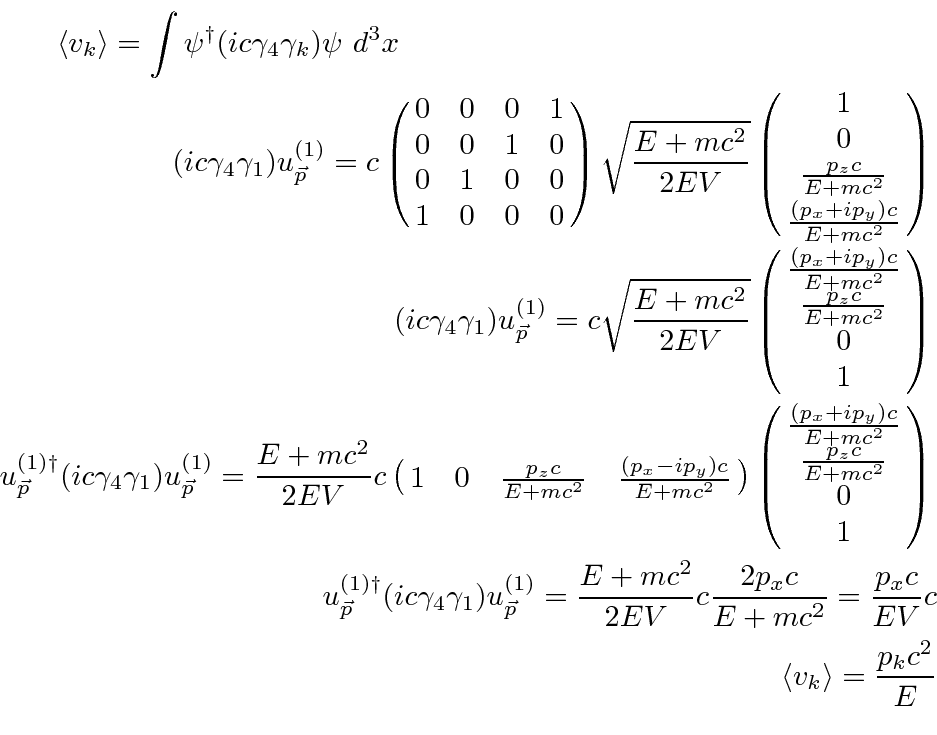
The expected value of a component of the velocity exhibits strange behavior when negative and positive energy states are mixed. Sakurai (equation 3.253) computes this. Note that we use the fact that $u^{(3,4)}$ have ``negative energy''.

$$\begin{eqnarray*} \langle v_k\rangle=\int\psi^\dagger (ic\gamma_4\gamma_k)\psi ... ...mma_k u^{(r')}_{\vec{p}} e^{2i\vert E\vert t/\hbar}\right] \\ \end{eqnarray*}$$

The last sum which contains the cross terms between negative and positive energy represents extremely high frequency oscillations in the expected value of the velocity, known as Zitterbewegung. The expected value of the position has similar rapid oscillations.

The Zitterbewegung again keeps electrons from being well localized in a deep potential raising the energy of s states. Its effect is already included in our calculation as it is the source of the Darwin term.