The Electromagnetic Field Tensor

The transformation of electric and magnetic fields under a Lorentz boost we established even before Einstein developed the theory of relativity. We know that E-fields can transform into B-fields and vice versa. For example, a point charge at rest gives an Electric field. If we boost to a frame in which the charge is moving, there is an Electric and a Magnetic field. This means that the E-field cannot be a Lorentz vector. We need to put the Electric and Magnetic fields together into one (tensor) object to properly handle Lorentz transformations and to write our equations in a covariant way.

The simplest way and the correct way to do this is to make the Electric and Magnetic fields components of a rank 2 (antisymmetric) tensor.

$$F_{\mu\nu}=\pmatrix{ 0 & B_z & -B_y & -iE_x \... ... & B_x & -iE_y \cr B_y & -B_x & 0 & -iE_z \cr iE_x & iE_y & iE_z & 0}$$

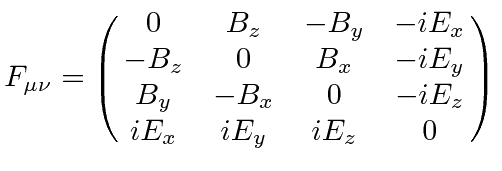The fields can simply be written in terms of the vector potential, (which is a Lorentz vector) $A_\mu=(\vec{A},i\phi)$.

$$F_{\mu\nu}={\partial A_\nu\over\partial x_\mu}-{\partial A_\mu\over\partial x_\nu}$$

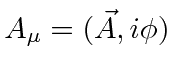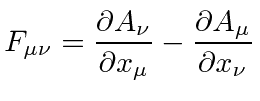Note that this is automatically antisymmetric under the interchange of the indices. As before, the first two (sourceless) Maxwell equations are automatically satisfied for fields derived from a vector potential. We may write the other two Maxwell equations in terms of the 4-vector $j_\mu=(\vec{j},ic\rho)$.

$${\partial F_{\mu\nu}\over\partial x_\nu}={j_\mu\over c}$$

Which is why the T-shirt given to every MIT freshman when they take Electricity and Magnetism should say

``... and God said 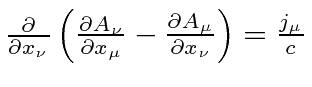${\partial\over\partial x_\nu}\left({\partial A_\nu\over\partial x_\mu}-{\partial A_\mu\over\partial x_\nu}\right) ={j_\mu\over c}$ and there was light.''

Of course he or she hadn't yet quantized the theory in that statement.

For some peace of mind, lets verify a few terms in the equations. Clearly all the diagonal terms in the field tensor are zero by antisymmetry. Lets take some example off-diagonal terms in the field tensor, checking the (old) definition of the fields in terms of the potential.

$$\begin{eqnarray*} \vec{B}&=&\vec{\nabla}\times \vec{A} \\ \vec{E}&=&-\vec{\nabl... ...artial x_i}+{1\over c}{\partial A_i\over\partial t}\right) =iE_i \end{eqnarray*}$$

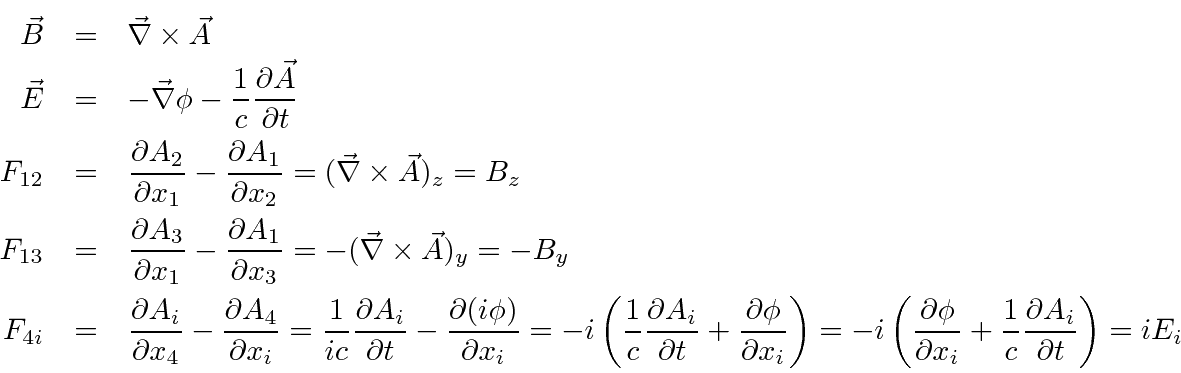
Lets also check what the Maxwell equation says for the last row in the tensor.

$$\begin{eqnarray*} {\partial F_{4\nu}\over\partial x_\nu}&=&{j_4\over c} \\ {\pa... ...over\partial x_i}&=&\rho \\ \vec{\nabla}\cdot\vec{E}&=&\rho \\ \end{eqnarray*}$$

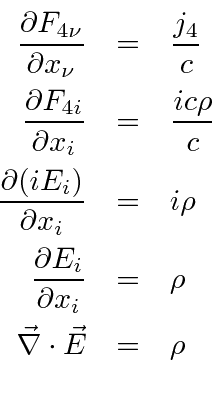
We will not bother to check the Lorentz transformation of the fields here. Its right.