Next: Phenomena of Radiation Theory Up: Lifetime and Line Width Previous: Lifetime and Line Width Contents
Collision broadening occurs when excited atoms or molecules have a large probability to change state when they collide with other atoms or molecules. If this is true, and it usually is, the mean time to collision is an important consideration when we are assessing the lifetime of a state. If the mean time between collisions is less than the lifetime, then the line-width will be dominated by collision broadening.
An atom or molecule moving through a gas sweeps through a volume per second proportional to its cross section
![]() and velocity.
The number of collisions it will have per second is then
and velocity.
The number of collisions it will have per second is then
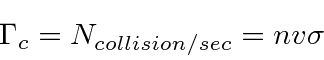

Doppler broadening is a simple non-quantum effect. We know that the frequency of photons is shifted if the source is moving - shifted higher if the source is moving toward the detector, and shifted lower if it is moving away.

Finally, we should be aware of the effect of recoil. When an atom emits a photon, the atom must recoil to conserve momentum. Because the atom is heavy, it can carry a great deal of momentum while taking little energy, still the energy shift due to recoil can be bigger than the natural line width of a state. The photon energy is shifted downward compared to the energy difference between initial and final atomic states. This has the consequence that a photon emitted by an atom will not have the right energy to be absorbed by another atom, raising it up to the same excited state that decayed. The same recoil effect shifts the energy need to excite a state upward. Lets do the calculation for Hydrogen.
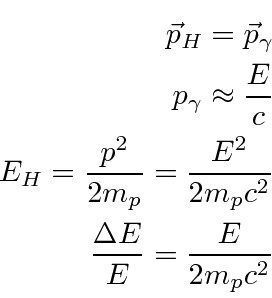
Jim Branson 2013-04-22