Next: Vibrational States Up: Molecular Physics Previous: Importance of Unpaired Valence Contents
There is an axis of symmetry for diatomic molecules.
This means
![]() commutes with
commutes with
![]() and
and
![]() is a good quantum number.
The different
is a good quantum number.
The different
![]() states, we have seen, have quite different shapes
therefore bond differently.
Imagine that a valence electron is in a
states, we have seen, have quite different shapes
therefore bond differently.
Imagine that a valence electron is in a
![]() state.
The
state.
The
 are called molecular orbitals
are called molecular orbitals
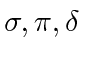 respectively.
Each has a bonding and an antibonding state.
respectively.
Each has a bonding and an antibonding state.
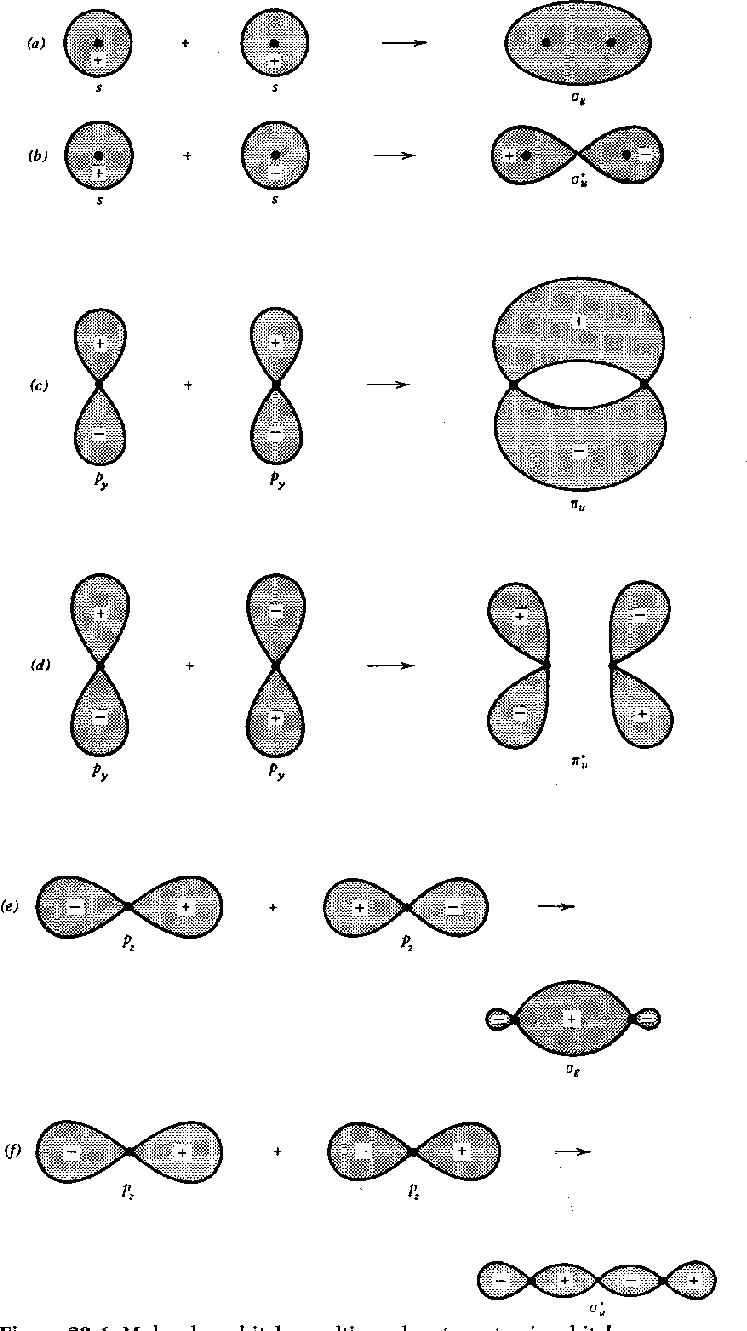
Pictures of molecular orbitals are shown for
![]() and
and
![]() states in the following
figure.
Both bonding and antibonding orbitals are shown first as atomic states then
as molecular.
The antibonding states are denoted by a *.
states in the following
figure.
Both bonding and antibonding orbitals are shown first as atomic states then
as molecular.
The antibonding states are denoted by a *.
Jim Branson 2013-04-22