- Find the allowed total spin states of two spin 1 particles.
Explicitly write out the 9 states which are eigenfunctions of
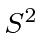 and
and 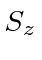 .
.
- The Hamiltonian of a spin system is given by
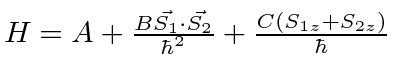 .
Find the eigenvalues and eigenfunctions of the system of two particles
(a) when both particles have spin
.
Find the eigenvalues and eigenfunctions of the system of two particles
(a) when both particles have spin 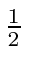 ,
(b) when one particle has spin
,
(b) when one particle has spin 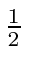 and the other spin 1.
What happens in (a) when the two particles are identical?
and the other spin 1.
What happens in (a) when the two particles are identical?
- Consider a system of two spinless identical particles.
Show that the orbital angular momentum of their relative motion
can only be even.
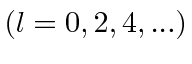 Show by direct calculation that, for the triplet spin states of
two spin
Show by direct calculation that, for the triplet spin states of
two spin 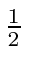 particles,
particles,
 for all allowed
for all allowed 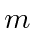 .
Show that for the singlet state
.
Show that for the singlet state
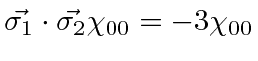 .
.
- A deuteron has spin 1. What are the possible spin and
total angular momentum states of two deuterons.
Include orbital angular momentum and assume the two particles are
identical.
- The state of an electron is given by
![$\psi=R(r)[\sqrt{1\over 3}Y_{10}(\theta,\phi)\chi_+ +\sqrt{2\over 3}Y_{11}(\theta,\phi)\chi_-]$](img2921.png) .
Find the possible values and the probabilities
of the
.
Find the possible values and the probabilities
of the 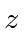 component of the electron's
total angular momentum.
Do the same for the total angular momentum squared.
What is the probability density for finding an electron with
spin up at
component of the electron's
total angular momentum.
Do the same for the total angular momentum squared.
What is the probability density for finding an electron with
spin up at
 ?
What is it for spin down?
What is the probability density independent of spin?
(Do not leave your answer in terms of spherical harmonics.)
?
What is it for spin down?
What is the probability density independent of spin?
(Do not leave your answer in terms of spherical harmonics.)
- The
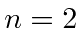 states of hydrogen have an 8-fold degeneracy due
to the various
states of hydrogen have an 8-fold degeneracy due
to the various 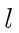 and
and 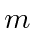 states allowed and the two spin states
of the electron.
The spin orbit interaction partially breaks the degeneracy by adding
a term to the Hamiltonian
states allowed and the two spin states
of the electron.
The spin orbit interaction partially breaks the degeneracy by adding
a term to the Hamiltonian
 .
Use first order perturbation theory to find how the degeneracy is broken under
the full Hamiltonian and write the approximate energy eigenstates in terms of
.
Use first order perturbation theory to find how the degeneracy is broken under
the full Hamiltonian and write the approximate energy eigenstates in terms of
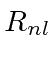 ,
, 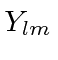 , and
, and  .
.
- The nucleus of a deuterium (A=2 isotope of H) atom is found to have spin 1.
With a neutral atom, we have three angular momenta to add, the nuclear spin,
the electron spin, and the orbital angular momentum. Define
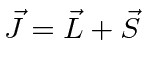 in the usual way and
in the usual way and
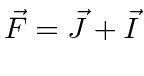 where
where 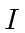 denotes the nuclear spin operator.
What are the possible quantum numbers
denotes the nuclear spin operator.
What are the possible quantum numbers 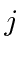 and
and 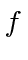 for an atom in the ground state?
What are the possible quantum numbers for an atom in the 2p state?
for an atom in the ground state?
What are the possible quantum numbers for an atom in the 2p state?
Jim Branson
2013-04-22
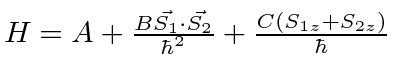 .
Find the eigenvalues and eigenfunctions of the system of two particles
(a) when both particles have spin
.
Find the eigenvalues and eigenfunctions of the system of two particles
(a) when both particles have spin 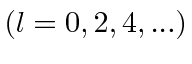 Show by direct calculation that, for the triplet spin states of
two spin
Show by direct calculation that, for the triplet spin states of
two spin  for all allowed
for all allowed 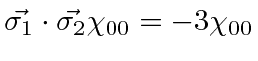 .
.
![$\psi=R(r)[\sqrt{1\over 3}Y_{10}(\theta,\phi)\chi_+ +\sqrt{2\over 3}Y_{11}(\theta,\phi)\chi_-]$](img2921.png) .
Find the possible values and the probabilities
of the
.
Find the possible values and the probabilities
of the  ?
What is it for spin down?
What is the probability density independent of spin?
(Do not leave your answer in terms of spherical harmonics.)
?
What is it for spin down?
What is the probability density independent of spin?
(Do not leave your answer in terms of spherical harmonics.)
 .
Use first order perturbation theory to find how the degeneracy is broken under
the full Hamiltonian and write the approximate energy eigenstates in terms of
.
Use first order perturbation theory to find how the degeneracy is broken under
the full Hamiltonian and write the approximate energy eigenstates in terms of
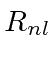 ,
, 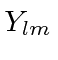 , and
, and 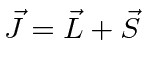 in the usual way and
in the usual way and
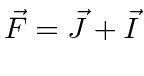 where
where