Next: Examples Up: Derivations and Computations Previous: 1D Model of a Contents
We are working with the periodic potential
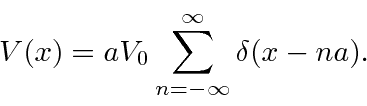
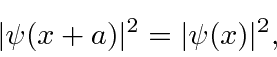

The general solution in the region
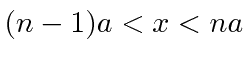 is
is
![\begin{displaymath}\bgroup\color{black} \psi_n(x)=A_n\sin(k[x-na])+B_n\cos(k[x-na]) \egroup\end{displaymath}](img1303.png)
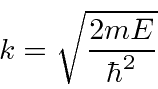
Now lets look at the boundary conditions at
![]() .
Continuity of the wave function gives
.
Continuity of the wave function gives
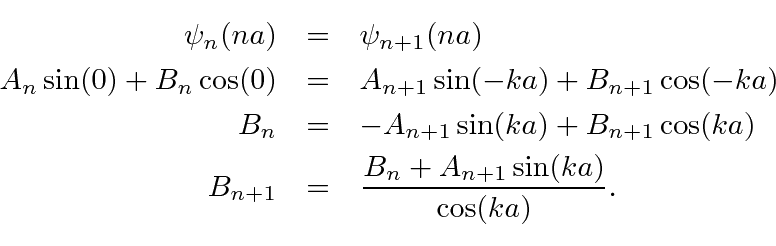
The discontinuity in the first derivative is
![\begin{eqnarray*}
\left.{d\psi_{n+1}\over dx}\right\vert _{na}
-\left.{d\psi_{n...
...A_{n+1}\cos(ka)+B_{n+1}\sin(ka)-A_n]={2maV_0\over\hbar^2}B_n \\
\end{eqnarray*}](img1387.png)
Substituting
 from the first equation
from the first equation
![\begin{eqnarray*}
k[A_{n+1}\cos(ka)+[B_n+A_{n+1}\sin(ka)]\tan(ka)-A_n]={2maV_0\o...
...+1}={2maV_0\over\hbar^2k}B_n\cos(ka)-B_n\sin(ka)+A_n\cos(ka) \\
\end{eqnarray*}](img1389.png)
Plugging this equation for
 back into the equation above for
back into the equation above for
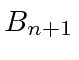 we get
we get
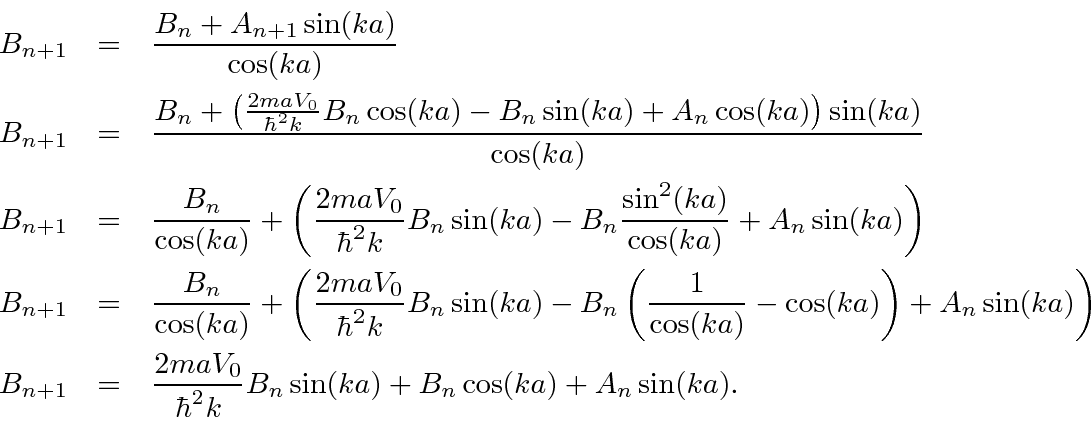
We now have two pairs of equations for the
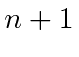 coefficients in terms of the
coefficients in terms of the
![]() coefficients.
coefficients.
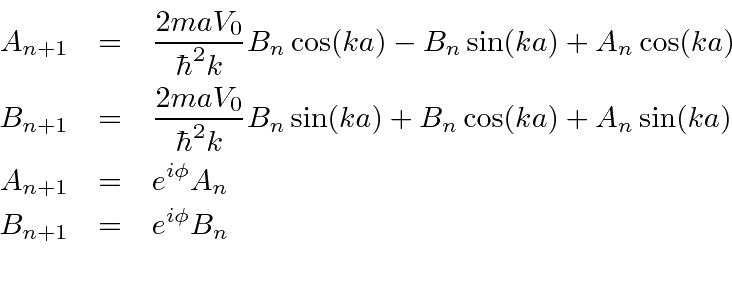
Using the second pair of equations to eliminate the
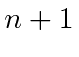 coefficients, we have
coefficients, we have

Now we can eliminate all the coefficients.

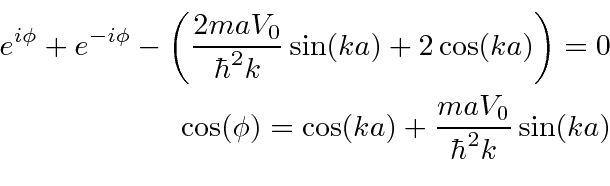
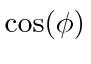 can only take on values between -1 and 1, there are allowed bands of
can only take on values between -1 and 1, there are allowed bands of
Jim Branson 2013-04-22