Next: Positronium Up: Examples Previous: Hydrogen in a Strong Contents
We continue on as in the last section but work in the states of
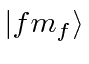 .
The matrix for
.
The matrix for
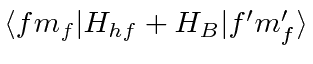 is
is
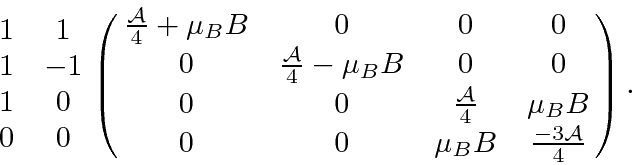
The top part is already diagonal so we only need to work in bottom right 2 by 2 matrix, solving the eigenvalue problem.
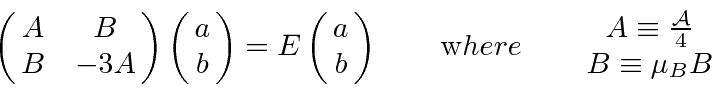
Setting the determinant equal to zero, we get
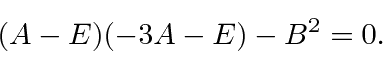
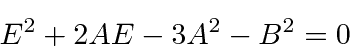

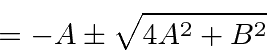
The eigenvalues for the
 states, which mix differently as a function
of the field strength, are
states, which mix differently as a function
of the field strength, are
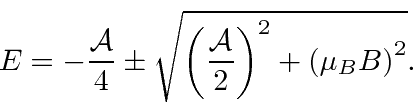
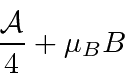
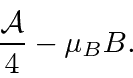
Jim Branson 2013-04-22