Next: Homework Problems Up: Derivations and Computations Previous: Derivations and Computations Contents
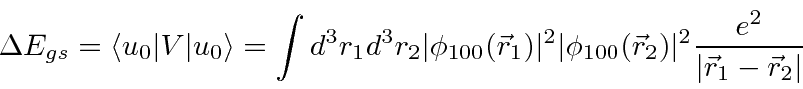
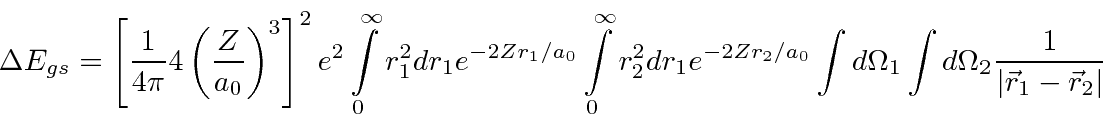

Do the
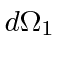 integral and prepare the other.
integral and prepare the other.

The angular integrals are not hard to do.
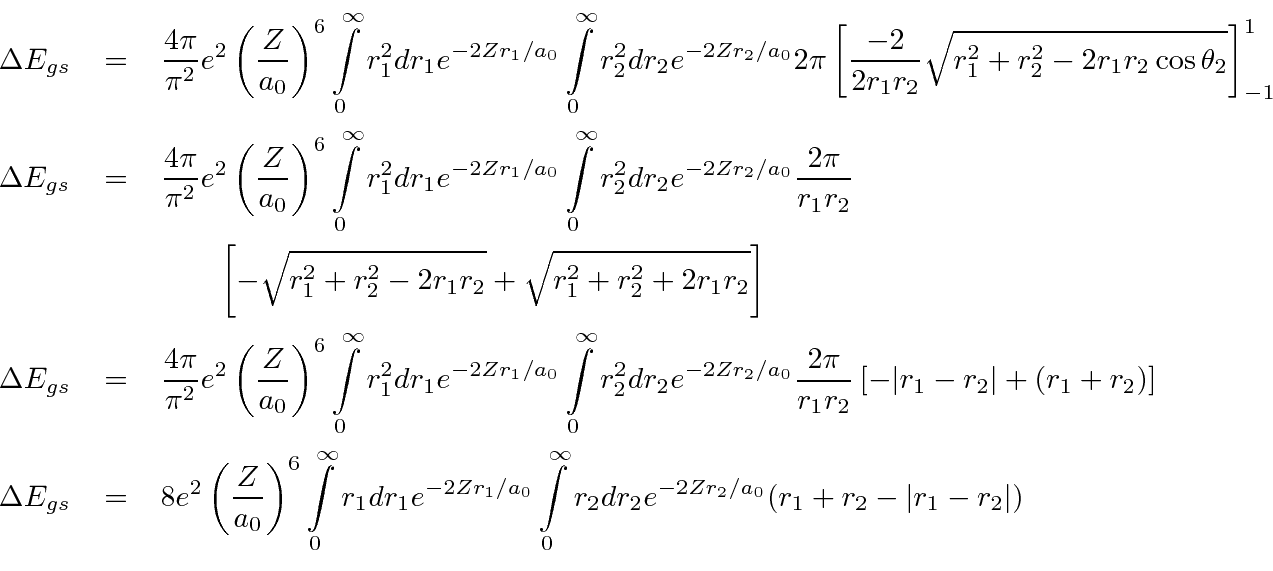
We can do the integral for
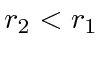 and simplify the expression.
Because of the symmetry between
and simplify the expression.
Because of the symmetry between
![]() and
and
![]() the rest of the integral
just doubles the result.
the rest of the integral
just doubles the result.
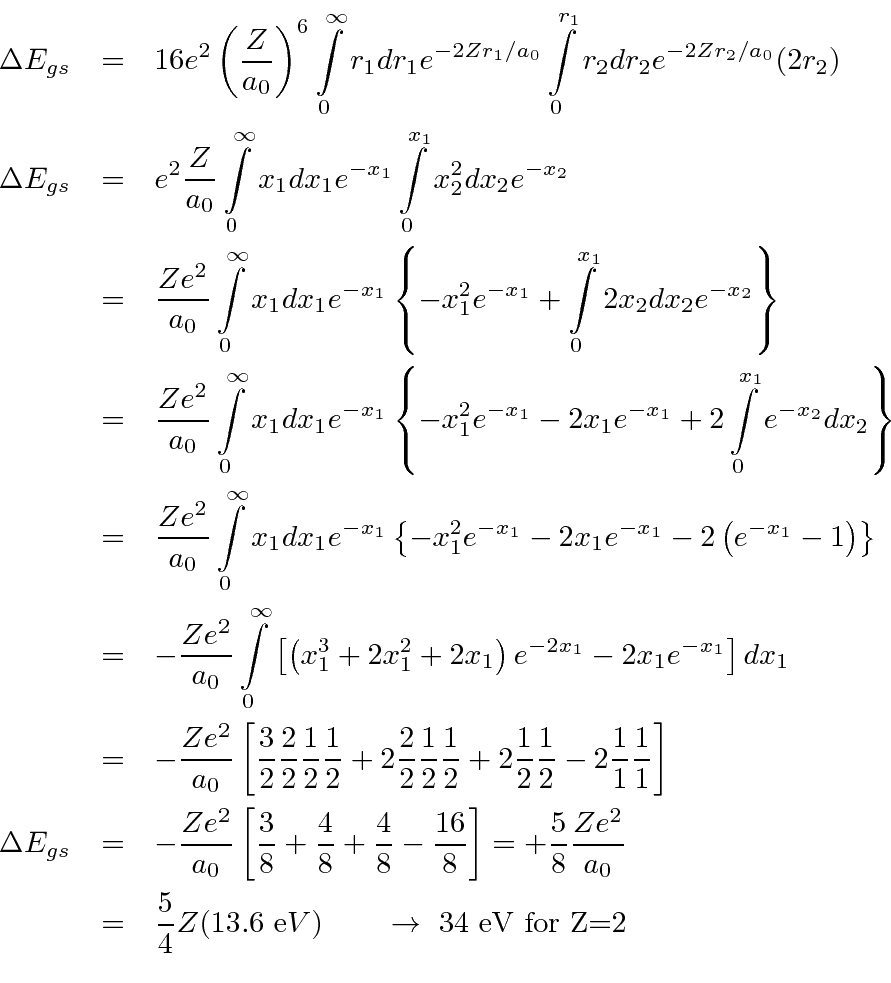
Jim Branson 2013-04-22