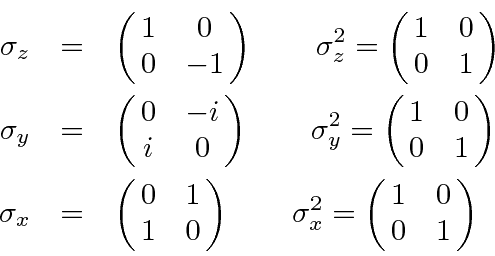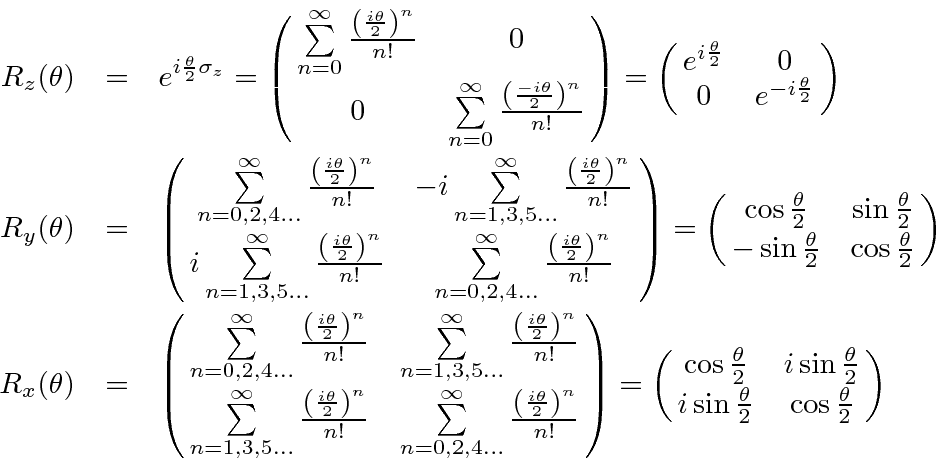Derive Spin 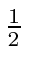${1\over 2}$ Rotation Matrices *

In section 18.11.3, we derived the expression for the rotation operator for orbital angular momentum vectors. The rotation operators for internal angular momentum will follow the same formula.

$$\begin{eqnarray*} R_z(\theta)&=&e^{i\theta S_z\over\hbar}=e^{i{\theta\over 2}\si... ...{n=0}^\infty {\left({i\theta\over 2}\right)^n\over n!}\sigma_j^n \end{eqnarray*}$$

We now can compute the series by looking at the behavior of $\sigma_j^n$.

$$\begin{eqnarray*} \sigma_z&=&\pmatrix{1 & 0\cr 0 & -1} \qquad \sigma_z^2=\pmatri... ...atrix{0 & 1\cr 1 & 0} \qquad \sigma_x^2=\pmatrix{1 & 0\cr 0 & 1} \end{eqnarray*}$$

Doing the sums

$$\begin{eqnarray*} R_z(\theta)&=&e^{i{\theta\over 2}\sigma_z}= \pmatrix{\sum\li... ...\theta\over 2} \cr i\sin{\theta\over 2} & \cos{\theta\over 2} } \end{eqnarray*}$$

Note that all of these rotation matrices become the identity matrix for rotations through 720 degrees and are minus the identity for rotations through 360 degrees.